104.二叉树的最大深度
力扣题目链接(opens new window)
给定一个二叉树,找出其最大深度。
二叉树的深度为根节点到最远叶子节点的最长路径上的节点数。
说明: 叶子节点是指没有子节点的节点。
示例: 给定二叉树 [3,9,20,null,null,15,7],

返回它的最大深度 3 。
#递归法
本题可以使用前序(中左右),也可以使用后序遍历(左右中),使用前序求的就是深度,使用后序求的是高度。
而根节点的高度就是二叉树的最大深度,所以本题中我们通过后序求的根节点高度来求的二叉树最大深度。
这一点其实是很多同学没有想清楚的,很多题解同样没有讲清楚。
我先用后序遍历(左右中)来计算树的高度。
- 确定递归函数的参数和返回值:参数就是传入树的根节点,返回就返回这棵树的深度,所以返回值为int类型。
代码如下:
1
| int getdepth(treenode* node)
|
- 确定终止条件:如果为空节点的话,就返回0,表示高度为0。
代码如下:
1
| if (node == NULL) return 0;
|
- 确定单层递归的逻辑:先求它的左子树的深度,再求的右子树的深度,最后取左右深度最大的数值 再+1 (加1是因为算上当前中间节点)就是目前节点为根节点的树的深度。
代码如下:
1
2
3
4
| int leftdepth = getdepth(node->left);
int rightdepth = getdepth(node->right);
int depth = 1 + max(leftdepth, rightdepth);
return depth;
|
所以整体c++代码如下:
1
2
3
4
5
6
7
8
9
10
11
12
13
| class solution {
public:
int getdepth(treenode* node) {
if (node == NULL) return 0;
int leftdepth = getdepth(node->left);
int rightdepth = getdepth(node->right);
int depth = 1 + max(leftdepth, rightdepth);
return depth;
}
int maxdepth(treenode* root) {
return getdepth(root);
}
};
|
代码精简之后c++代码如下:
1
2
3
4
5
6
7
| class solution {
public:
int maxdepth(treenode* root) {
if (root == null) return 0;
return 1 + max(maxdepth(root->left), maxdepth(root->right));
}
};
|
精简之后的代码根本看不出是哪种遍历方式,也看不出递归三部曲的步骤,所以如果对二叉树的操作还不熟练,尽量不要直接照着精简代码来学。
本题当然也可以使用前序,代码如下:(充分表现出求深度回溯的过程)
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
| class solution {
public:
int result;
void getdepth(treenode* node, int depth) {
result = depth > result ? depth : result;
if (node->left == NULL && node->right == NULL) return ;
if (node->left) {
depth++;
getdepth(node->left, depth);
depth--;
}
if (node->right) {
depth++;
getdepth(node->right, depth);
depth--;
}
return ;
}
int maxdepth(treenode* root) {
result = 0;
if (root == NULL) return result;
getdepth(root, 1);
return result;
}
};
|
可以看出使用了前序(中左右)的遍历顺序,这才是真正求深度的逻辑!
注意以上代码是为了把细节体现出来,简化一下代码如下:
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
| class solution {
public:
int result;
void getdepth(treenode* node, int depth) {
result = depth > result ? depth : result;
if (node->left == NULL && node->right == NULL) return ;
if (node->left) {
getdepth(node->left, depth + 1);
}
if (node->right) {
getdepth(node->right, depth + 1);
}
return ;
}
int maxdepth(treenode* root) {
result = 0;
if (root == 0) return result;
getdepth(root, 1);
return result;
}
};
|
#迭代法
使用迭代法的话,使用层序遍历是最为合适的,因为最大的深度就是二叉树的层数,和层序遍历的方式极其吻合。
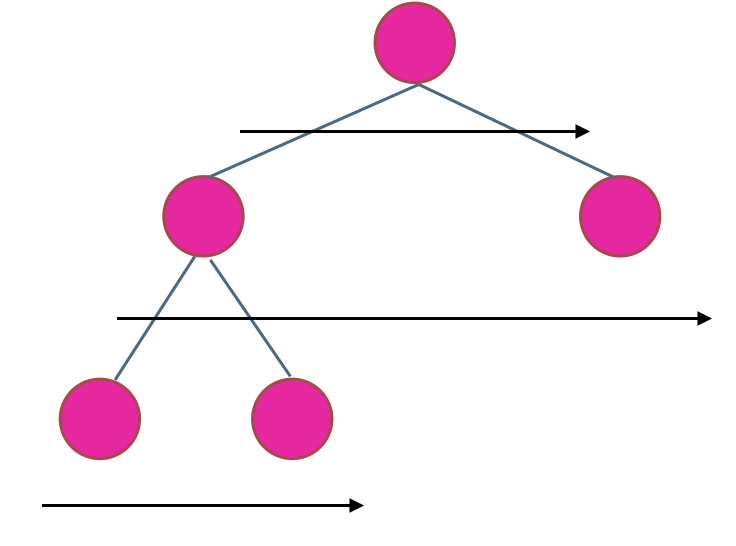
在二叉树中,一层一层的来遍历二叉树,记录一下遍历的层数就是二叉树的深度,如图所示:

所以这道题的迭代法就是一道模板题,可以使用二叉树层序遍历的模板来解决的。
如果对层序遍历还不清楚的话,可以看这篇:二叉树:层序遍历登场!(opens new window)
c++代码如下:
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
| class solution {
public:
int maxdepth(treenode* root) {
if (root == NULL) return 0;
int depth = 0;
queue<treenode*> que;
que.push(root);
while(!que.empty()) {
int size = que.size();
depth++;
for (int i = 0; i < size; i++) {
treenode* node = que.front();
que.pop();
if (node->left) que.push(node->left);
if (node->right) que.push(node->right);
}
}
return depth;
}
};
|
rust:
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
| impl Solution {
pub fn max_depth(root: Option<Rc<RefCell<TreeNode>>>) -> i32 {
if root.is_none(){
return 0;
}
let mut max_depth: i32 = 0;
let mut stack = vec![root.unwrap()];
while !stack.is_empty() {
let num = stack.len();
for _i in 0..num{
let top = stack.remove(0);
if top.borrow_mut().left.is_some(){
stack.push(top.borrow_mut().left.take().unwrap());
}
if top.borrow_mut().right.is_some(){
stack.push(top.borrow_mut().right.take().unwrap());
}
}
max_depth+=1;
}
max_depth
}
|
那么我们可以顺便解决一下n叉树的最大深度问题
#559.n叉树的最大深度
力扣题目链接(opens new window)
给定一个 n 叉树,找到其最大深度。
最大深度是指从根节点到最远叶子节点的最长路径上的节点总数。
例如,给定一个 3叉树 :

我们应返回其最大深度,3。
思路:
依然可以提供递归法和迭代法,来解决这个问题,思路是和二叉树思路一样的,直接给出代码如下:
#递归法
c++代码:
1
2
3
4
5
6
7
8
9
10
11
| class solution {
public:
int maxdepth(node* root) {
if (root == 0) return 0;
int depth = 0;
for (int i = 0; i < root->children.size(); i++) {
depth = max (depth, maxdepth(root->children[i]));
}
return depth + 1;
}
};
|
#迭代法
依然是层序遍历,代码如下:
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
| class solution {
public:
int maxdepth(node* root) {
queue<node*> que;
if (root != NULL) que.push(root);
int depth = 0;
while (!que.empty()) {
int size = que.size();
depth++;
for (int i = 0; i < size; i++) {
node* node = que.front();
que.pop();
for (int j = 0; j < node->children.size(); j++) {
if (node->children[j]) que.push(node->children[j]);
}
}
}
return depth;
}
};
|
#其他语言版本
java代码如下:
递归法:
1
2
3
4
5
6
| public int maxDepth(TreeNode root) {
if(root == null) return 0;
int left = maxDepth(root.left);
int right = maxDepth(root.right);
return 1 + Math.max(left,right);
}
|
迭代法:
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
| public int maxDepth(TreeNode root) {
if(root == null) return 0;
Queue<Integer> que = new LinkedList<>();
que.offer(root);
int res = 0;
while(!que.isEmpty()) {
res++;
int size = que.size();
for(int i=0;i<size;i++) {
TreeNode node = que.poll();
if(node.left != null) que.offer(node.left);
if(node.right != null) que.offer(node.right);
}
}
return res;
}
|
n叉树的最大深度